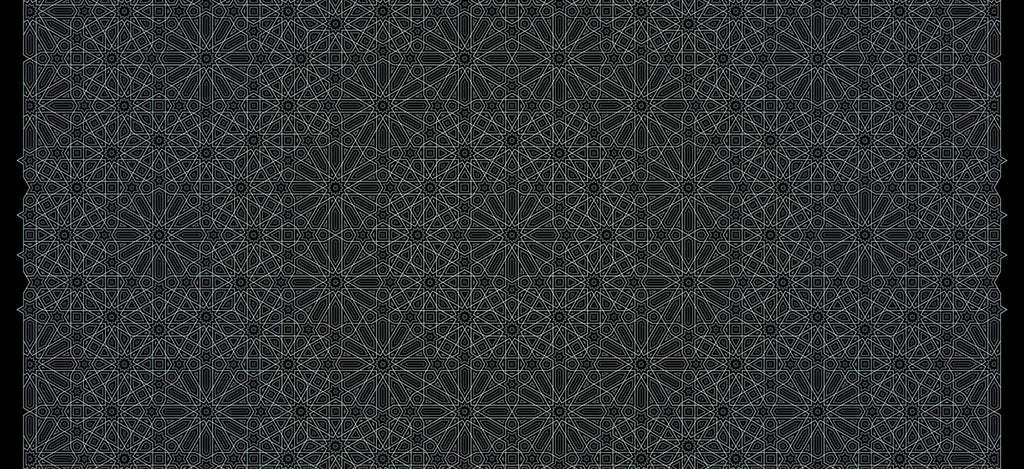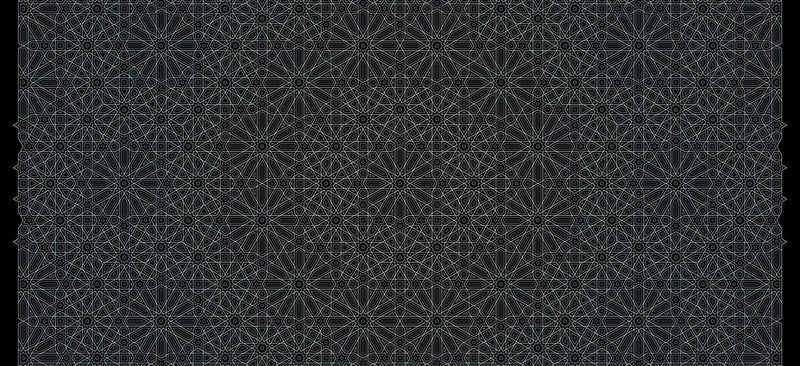
I’ve been completely obsessed with exploring two dimensional geometry for about ten years now, and wanted to take this opportunity to dive deep into my most beloved shape of all, the 12 pointed star. Recently I completed my largest work to date, titled “Expansion,” which is constructed entirely out of the 12 pointed star and the shapes embedded within it. We will examine the 12 pointed star, then take what we’ve learned and apply it to decoding the macro and micro / self repeating / fractal relationships hidden within my piece. Warning – please do not read any further unless you are prepared to have your mind sufficiently blasted and then reassembled again into an infinite interdimensional grid of precisely balanced geometric chaos and order.
…..jk lol

I have a long history with this shape, and it never fails to amaze me in how many different possibilities lie within it. My relationship with the 12 pointed star began around 2009, when I was beginning to tinker with geometry. This was before I began using Adobe Illustrator as my sole method of creation, and I was drafting everything with a compass, straight edge and square (the traditional tools used for geometric construction). If you are looking to explore geometry, definitely start with these basic yet essential tools. I moved onto Illustrator to speed up the process, and now spend much less time drafting and more time exploring.
Through my early exploration, I came across the 12 pointed star by drawing a standard hexagram, then drawing another hexagram on top, only rotated a quarter turn (90*). This essentially doubles the hexagram, giving way to a 12 pointed star. It took me a few more years to realize the shape was incomplete without the extended rays (shown in figure 6 below).. But before we get too far in the weeds, let’s take a moment to absorb this diagram I put together to visually demonstrate the different shapes and relationships hidden within the magnificent 12 pointed star, as well as methods of construction.

Ok, so now that we have the diagram to refer to, let’s dissect what makes this shape so special. The 12 pointed star combines systems of 3, 4 and 6, which can be visually represented as triangles (3 sides), squares (4 sides), and hexagons (6 sides). What makes this significant is that these systems don’t tile well together without the presence of 12. By “multiplying” 3 x 4, we are given the magic 12. Now again to what makes this significant – triangles (3) tile in an isometric grid, squares (4) tile in a checkerboard grid, while hexagons (6) tile in a honeycomb grid. Refer to the examples below, and compare their differences..

By working with the twelve pointed star, you can essentially combine aspects of each of these radically different tiling systems into the same construction. This is evidenced below in the series of hidden shapes, extracted from my piece “Expansion.” I have simplified the artwork (figure 1) in order for the hidden relationships to be more apparent. Now that we have laid the ground work, you will easily be able to identify each of the shapes highlighted, and understand the significance of them all working together in harmony. Below the gallery are explanations of each slide.
To start things off, in figures 2 and 3 I have highlighted the hidden 12 pointed stars within expansion. Notice how in #2 the rays extend to the outer edge of the canvas, and on #3 the rays extend to the corners of the canvas. Also examine the line intersections in each of the slides – they usually correspond with smaller 12 pointed stars, hexagrams, and squares.. Interesting, very interesting indeed!
Figures 4 and 5 show the hidden 12 sided polygons (known as dodecagons) within “Expansion”. These two slides present a recurring pattern within macro “hidden shapes” and other dual level geometry. The first slide (#4) shows all of the concentric repeating shapes, and the second slide (#5) shows how the shapes tile outward. Something to note with #5 is how the central 12 sided polygon is surrounded by twelve 12 sided polygons… This is an example of self repeating patterns, otherwise known as fractals, where the characteristics of the pattern in the micro scale are echoed in the macro scale. Another point of interest within #4 and #5 is how the shapes flow into the outer edge of the canvas.
Figures 6 and 7 highlight a seamless isometric grid over the entire pattern. The two slides contain the same geometry, but are both included to demonstrate how the same pattern is present at both 90* (triangles pointing up and down in #6) and 0* (triangles pointing side to side in #7). Notice how the lines within these slides intersect over either a hexagram or 12 pointed star, which makes sense considering they both contain a multiple of 3 and 6. But wait, there’s more..
Figures 8 and 9 show the hexagrams and doubled hexagrams present within “Expansion”. I find #9 to be particularly beautiful, with all of the squares created in the negative space from the overlapping hexagrams, yet another example of triangles and squares working together within this pattern.
Figures 10 and 11 demonstrate the presence of hexagons within the pattern. Like #6 and #7, these hidden macro shapes are also present if you rotate them 90*. It is particularly interesting seeing the seamless hexagonal pattern in #11 – the overall geometric composition of this piece follows a radial tiling construction, as opposed to a standard tiling pattern, like I demonstrated earlier through isometric / checkerboard / honeycomb grids. These standard grids have no defined center, whereas radial tilings all start from a central point, and infinitely tile outwards from there. So to see a static hexagonal, square, or isometric grid seamlessly laid over a radial tiling further demonstrates the interconnected and fractal nature of geometry.
Slides 12 – 17 show the hidden squares within the piece. The first three slides (12 – 14) show the different radial and standard tilings present, while the following three slides demonstrate how each of these tilings are still present when rotated 30* or 60.* This is again further demonstration of how the square, hexagonal and triangular grids are all working together in unison. It makes sense that we could rotate these squares within the pattern, because 4 (square) x 3 = 12. In other words, there are three square orientations present within the pattern.
Figure 18 shows how the lines dividing the pattern’s symmetry (akin to pie slices) are also present within the hidden macro shapes. This slide also echoes the repeating radial quality of slides 3, 4, 8, 9, 10, and 12
Thank you for reading and happy trails!
-Kyle Carter
CogDut